
AP Calculus is a popular course for high schoolers, particularly those planning to pursue a college education. Hundreds of thousands of high schoolers each year study to obtain a passing/high score on the AP Calculus AB Exam so that they can “test out” of math distribution requirements in the early university years and save time and money.
In 2022, over 250,000 of the 1.2 million students taking AP exams took the AP Calculus AB exam. This places it among the top 4 most popular AP exams. If you are interested in taking the AP Calculus AB exam, whether you have taken the class or are planning to self-study, read on for a breakdown of the test and advice on how to best prepare for it.
The purpose of the AP Calculus AB exam is to test your knowledge of specific “big concepts” that you have learned either through taking the AP Calculus AB course or through self-study.
The “big concepts” of AB Calculus, as defined by College Board, are:
With regard to limits, students should be comfortable with computing limits, including one-sided limits, limits at infinity, the limit of a sequence, and infinite limits. The exam will also test each student’s ability to estimate the limit of a function at a point and apply limits to understand the behavior of a function near a point.
With regard to derivatives, students should be comfortable with finding the slope of a tangent line to a graph at a point and using a graph to determine whether a function is increasing or decreasing. Students should also be able to find concavity and find extreme values. Additionally, the exam will require students to solve problems involving rectilinear motion.
Finally, with regard to integrals, students should be able to use various techniques and methods to approximate an integral. Students should also be familiar with area, volume, and motion applications of integrals, as well as with the use of the definite integral as an accumulation function.
The AP Calculus AB exam is one of the longest AP exams, clocking in at three hours and 15 minutes. It has two sections. The first section contains 45 multiple choice questions, spans one hour and 45 minutes, and accounts for 50% of your total score. The second section consists of six free response questions, spans one hour and 30 minutes, and accounts for the remaining 50% of your score.
Each section is divided into two parts, Part A and Part B. Students are permitted to use calculators during one part and not allowed to use them during the other.
Skill Assessed
Types of Question
Number of Questions
Scoring Weight
Section I, Part A
Multiple Choice without Graphing Calculator
Algebraic, exponential, logarithmic, trigonometric, and general types of functions
Section I, Part B
Multiple Choice with Graphing Calculator
Analytical, graphical, tabular, and verbal types of representations
Section II, Part A
Free Response with Graphing Calculator
Various types of functions and function representations and a roughly equal mix of procedural and conceptual tasks
Section II, Part B
Free Response without Graphing Calculator
Questions that incorporate a real-world context or scenario into the question
While taking the AP Calculus AB exam, you may use a scientific calculator on Part B of the multiple choice section and on Part A of the free response section. Your calculator should be able to plot the graph of a function within an arbitrary viewing window, find the zeros of functions, numerically calculate the derivative of a function, and numerically calculate the value of a definite integral. More information and a list of acceptable calculator models can be found in the official Calculator Policy .
Note: A calculator may not be used on questions on this part of the exam.

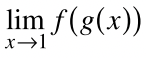
1. The graphs of the functions f and g are shown above. The value of is
Note: A graphing calculator is required for some questions on this part of the exam.
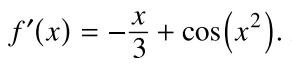
1. The derivative of the function f is given by
At what values of x does f have a relative minimum on the interval 0 < x < 3?
(A) 1.094 and 2.608

2. The second derivative of a function g is given by
Note: A graphing calculator is required for problems on this part of the exam.


1. Let R be the region in the first quadrant bounded by the graph of g , and let S be the region in the first quadrant between the graphs of f and g , as shown in the figure above. The region in the first quadrant bounded by the graph of f and the coordinate axes has area 12.142. The function g is given by and the function f is not explicitly given. The graphs of f and g intersect at the point (4,0).
(A) Find the area of S .
(B) A solid is generated when S is revolved about the horizontal line y = 5. Write, but do not evaluate, an expression involving one or more integrals that gives the volume of the solid.
(C) Region R is the base of an art sculpture. At all points in R at a distance x from the y -axis, the height of the sculpture is given by h(x) = 4 – x . Find the volume of the art sculpture.
Rochelle rode a stationary bicycle. The number of rotations per minute of the wheel of the stationary bicycle at time t minutes during Rochelle’s ride is modeled by a differentiable function r for 0 ≤ t ≤ 9 minutes. Values of r(t) for selected values of t are shown in the table above.
(A) Estimate r’ (4). Show the computations that lead to your answer. Indicate units of measure.
(B) Is there a time t , for 3 ≤ t ≤ 5 at which r(t) is 106 rotations per minute? Justify your answer.
(C) Use a left Riemann sum with the four subintervals indicated by the data in the table to approximate Using correct units, explain the meaning of in the context of the problem.
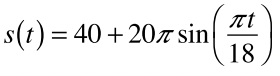
(D) Sarah also rode a stationary bicycle. The number of rotations per minute of the wheel of the stationary bicycle at time t minutes during Sarah’s ride is modeled by the function s , defined by for 0 ≤ t ≤ 9 minutes. Find the average number of rotations per minute of the wheel of the stationary bicycle for 0 ≤ t ≤ 9 minutes.
While many exam distributions fall along a bell curve, with the majority of students receiving a score of 3, the AP Calculus AB exam shows a flatter distribution. Simply put, many students do well and many students do poorly. In 2022:
This means that 55.6% of students who took the exam received a 3 or higher (typically considered passing).
Note: The credit you will receive for AP exam scores varies widely from school to school. For example, prestigious schools (and even prestigious programs at schools) might accept only a 4 or a 5 to receive course credit. Though a score of 3 is typically considered passing, it’s not always enough. You can use this search tool to see what scores will allow you to receive credit at a specific college or university.
Take a practice test to assess your initial knowledge of the material. It’s important to know where you are, so that you know how far you need to go.
Keep in mind that Calculus is an age-old study, so you can use practice tests from before you were even born and you’ll be assessing/learning just the same!
There are a couple of options for taking practice tests:
The 2012 exam has been openly published by the College Board and might be a good place to start. The College Board also has free response questions from the last few decades published online, though you should note that these are not complete assessments.
Once you have taken your formative assessment, score it to identify the areas you already understand and those in need of improvement.
Note: When grading the free response portion of the exam, make sure you grade yourself based on the rubric! Act like you are an AP scorer, scrutinizing and nitpicking every portion of your answer. The little points add up, and your area of improvement could very well be “needing to show my work.”
After taking your assessment, you should be able to identify areas that need improvement. These areas could be related to content—not knowing which technique should be used to approximate which type of integral or not understanding the relationship between concavity and limits on a graph. Alternatively, the areas you struggle with might have more to do with form—like struggling to read graphs or conceptualize tables.
Identify your areas for improvement, write them down, and focus on one area during each study session. Look over your mistakes and put in the work to understand them. Watch videos online about specific concepts, read sections in books about them, and talk to your friends and classmates about them.
Then do it again and again and again until the areas you struggle with become areas you excel in.
Some students choose to use commercial study guides when studying. This can be extremely beneficial, depending on your learning style. That said, if you choose to use a commercial study guide, use it in conjunction with your initial assessment. Study books are divided into sections organized around both big and small concepts. Don’t get stuck reading a guide front to back and don’t waste time on content that you have already mastered!
Lastly, you might consider looking into the free resources that are available online! For decades, AP teachers have been publicly posting complete study guides, review sheets, and test questions. Use these for your benefit.
Once you feel like you’ve mastered the concepts you initially struggled with, put them into action by answering some multiple choice practice questions.
The College Board provides a set of sample questions with scoring explanations . Additionally, the College Board Course Description includes many practice multiple choice questions along with explanations of their answers. As you go through these, try to keep track of which areas are still tripping you up, and go over those concepts again until you have a better grasp on them. Focus on understanding what each question is asking and keep a running list of any vocabulary that is still unfamiliar to you.
When you score your own formative assessment, you will notice that every step you take to arrive at a solution to a free response question must be clearly notated for the exam reader. Even if you use your calculator to solve an equation, compute a numerical derivative, or find a definite integral, write the equation, derivative, or integral first. Otherwise, you can lose little points—and little points add up!
The free response portion of the AP Calculus AB exam tests your ability to solve problems using an extended chain of reasoning. In most cases, an answer without supporting work will receive no credit. This means that, as you answer practice free response questions, you are not just practicing getting the right answer, but getting the right answer in the right way!
You can get a better understanding of the free response section’s scoring by reading scoring commentary from the Development Committee and authentic examples of student responses and their scoring explanations from previous exam administrations.
Every couple of weeks, when you are feeling confident or when you just want to see your progress, we recommend that you take another complete practice test. This will allow you to see which areas have improved the most and which areas still need improvement. Taking new practice tests at some interval will serve as a progress report of sorts.
In 2024, the AP Calculus AB Exam will be administered on Monday, May 13 at 8 AM local time. The day before, make sure you have everything you need, and then focus on getting a good night’s sleep. Studies show that being well-rested is far more likely to lead to improved performance than last-minute cramming!
While AP scores themselves don’t play a major role in the college admissions process, having AP classes on your transcript can be a crucial part of your application, especially at highly selective institutions. College admissions officers want to see that you enjoy challenging yourself intellectually, and that you’re capable of handling college-level coursework, and taking AP classes demonstrates both of those qualities.
The main benefit of scoring high on AP exams comes once you land at your dream school, as high scores can allow you to “test out” of entry-level requirements, often called GE requirements or distribution requirements. This will save you time and money.
If you’re starting to think about what schools you should apply to, we recommend that you use CollegeVine’s free chancing engine . This tool will consider your test scores, GPA, extracurriculars, and more, to calculate your chances of acceptance at various schools and to help you decide where to apply. It can also give you suggestions for how to boost your chances of acceptance—for example, by taking more AP classes in your junior or senior year.